Stable Distributions
2. Parameterisation of stable
distributions
[this page | pdf | references | back links]
Return
to Abstract and Contents
Next
page
2.1 As noted in Section 1 any
specific stable distributional form is characterised by four parameters 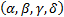 .
Nolan (2005)
notes that there are multiple definitions used in the literature regarding what
these parameters mean. He focuses there on two, which he denotes by
.
Nolan (2005)
notes that there are multiple definitions used in the literature regarding what
these parameters mean. He focuses there on two, which he denotes by 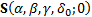 and
and
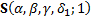 ,
that are differentiated according to the meaning given to
,
that are differentiated according to the meaning given to  . The
first is the one that he concentrates on, because it has better numerical
behaviour and intuitive meaning, but the second is more commonly used in the
literature. We call the former the ‘0-parameterisation’ and the latter the
‘1-parameterisation’ in these pages.
. The
first is the one that he concentrates on, because it has better numerical
behaviour and intuitive meaning, but the second is more commonly used in the
literature. We call the former the ‘0-parameterisation’ and the latter the
‘1-parameterisation’ in these pages.
2.2 In
either of these descriptions:
(a) 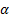 is the index
of the distribution, also known as the index of stability or characteristic
exponent, and must be in the range
is the index
of the distribution, also known as the index of stability or characteristic
exponent, and must be in the range 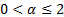 .
The constant
.
The constant  in
the formula in Section
1.3 must be of the form
in
the formula in Section
1.3 must be of the form 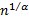 ;
;
(b) 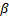 is the skewness
of the distribution and must be in the range
is the skewness
of the distribution and must be in the range 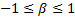 . If
. If 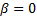 then
the distribution is symmetric, if
then
the distribution is symmetric, if 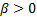 then it is skewed to the
right and if
then it is skewed to the
right and if 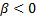 then it is skewed to the
left;
then it is skewed to the
left;
(c)  is a
scale parameter and can be any positive number; and
is a
scale parameter and can be any positive number; and
(d)  is a
location parameter, shifting the distribution right if
is a
location parameter, shifting the distribution right if 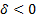 and
left if
and
left if 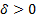 .
.
2.3In either description, the distributional form is
normally defined via the distribution’s characteristic function, i.e. the
(complex) function 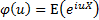 ,
where
,
where 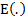 is
the expectation operator. Nolan (2005) uses the following definitions:
is
the expectation operator. Nolan (2005) uses the following definitions:
(a) A random
variable  is
is 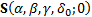 if
it has characteristic function
if
it has characteristic function
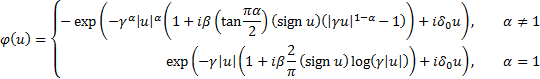
(b) A random variable  is
is 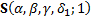 if
it has characteristic function
if
it has characteristic function
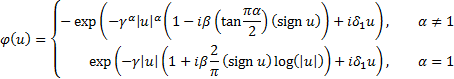
2.4 The
location parameters are related by:
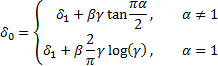
or
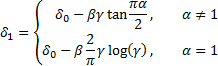
2.5 Nolan (2005)
notes that if 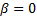 then the
0-parameterisation and the 1-parameterisation coincide. When
then the
0-parameterisation and the 1-parameterisation coincide. When 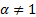 and
and 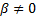 then
the parameterisations differ by a shift
then
the parameterisations differ by a shift 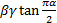 which
gets infinitely large as
which
gets infinitely large as  . Nolan argues that the
0-parameterisation is a better approach because it is jointly continuous in all
four parameters, but accepts that the 1-parameterisation is simpler
algebraically, so is unlikely to disappear from the literature.
. Nolan argues that the
0-parameterisation is a better approach because it is jointly continuous in all
four parameters, but accepts that the 1-parameterisation is simpler
algebraically, so is unlikely to disappear from the literature.
NAVIGATION LINKS
Contents | Prev | Next