/

Risk Attribution

3. Beta-adjusted attribution

[this page | pdf | references | back links]

Return to Abstract and Contents

Next page

 

3.1          A problem that arises with the above approach for traditional long-only portfolios is that such portfolios are typically benchmarked against market indices and often have ‘betas’, i.e. exposures to the market, which are close to one. This can make traditional risk decompositions versus the benchmark of such portfolios very sensitive to small changes in the amount of cash held within the portfolio (because cash has a beta of zero, i.e. substantially different to the benchmark’s beta of one), limiting the usefulness (or rather the stability) of the above decomposition for practical portfolio management. Better may be to decompose each instrument’s contribution to risk into a beta component and a remainder, with only the latter then subject to further decomposition in the usual manner.

 

3.2          Beta is a measure of how much a portfolio (or of an individual security) might be expected to rise or fall as the market (i.e. benchmark) rises or falls. A beta of 1 means that, all other things being equal, a 1 basis point rise or fall in the market leads to a corresponding 1 basis point rise or fall in the portfolio value. A beta of more than one means that all other things being equal the portfolio should rise or fall more than the corresponding rise or fall in the market, a beta of less than one means it should rise or fall less than the corresponding rise or fall in the market. Betas can, of course, be negative (e.g. a put option would typically have a negative beta, since it rises in value as the underlying falls). Long only portfolios typically have betas that are not too far from one, this being by definition the average beta of the relevant index being used as the benchmark for the long only portfolio.

 

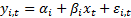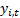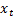3.3          Beta is benchmark specific, i.e. a stock with a given beta against one market index may have a different beta against a different market index. The terminology ‘beta’ arises because in effect we are ascribing a security’s (or an entire portfolio’s) return in a manner akin to a regression analysis in which  where  is the return on the i’th security in the t’th time period,  is the return on the market index in the t’th time period. Conventionally the intercept of this regression is typically referred to as the ‘alpha’ and the slope of this regression as the ‘beta’.

 

3.4          From an ex-ante risk perspective, the portfolio beta can be calculated as:

 

 

3.5          The active portfolio beta is then:

 

 

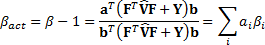
where we have decomposed the overall active beta into contributions from each individual (active) position, , where

 

 

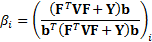
3.6          The marginal contribution to tracking error from a portfolio’s beta is then

 

 

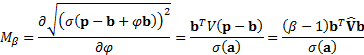
3.7          The portfolio’s overall active contribution to tracking error from its beta is therefore:

 

 

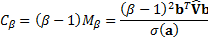
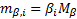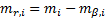3.8          We can apportion the marginal contribution to tracking error from the portfolio beta across individual securities using, say,  and thus identify the ‘residual’ (non-beta) element of each security’s marginal contribution to tracking error as, say, . The active ‘residual’ (non-beta) contribution to tracking error by security would then be .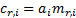

 

---

NAVIGATION LINKS
Contents | Prev | Next

---