The Student’s t distribution
[this page | pdf | back links]
The Student’s t distribution (more simply the t
distribution) arises when estimating the mean of a normally distributed
population when sample sizes are small and the population standard deviation is
unknown.
![[SmartChart]](I/StudentsTDistribution_files/image001.gif)
![[SmartChart]](I/StudentsTDistribution_files/image002.gif)
![[SmartChart]](I/StudentsTDistribution_files/image003.gif)
|
Distribution name
|
(Standard) Student’s
t distribution
|
|
Common notation
|
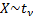
|
|
Parameters
|
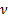 =
degrees of freedom ( =
degrees of freedom ( , usually , usually 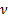 is
an integer although in some situations a non-integral is
an integer although in some situations a non-integral 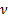 can
arise) can
arise)
|
|
Domain
|
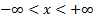
|
|
Probability density
function
|
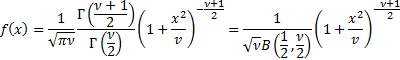
|
|
Cumulative distribution
function
|
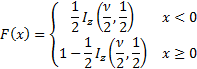
where 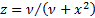
|
|
Mean
|

|
|
Variance
|
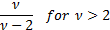
|
|
Skewness
|
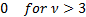
|
|
(Excess) kurtosis
|

|
|
Characteristic function
|
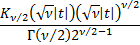
where 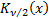 is a Bessel function is a Bessel function
|
|
Other comments
|
It is a special case of the generalised hyperbolic
distribution.
Its non-central moments if 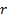 is
even and is
even and 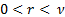 are: are:
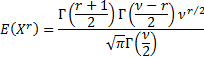
If 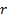 is even and is even and 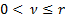 then then 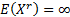 , if , if 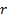 is
odd and is
odd and 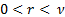 then then 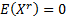 and if and if 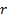 is
odd and is
odd and 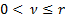 then then 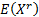 is undefined. is undefined.
|
Nematrian web functions
Functions relating to the above distribution may be accessed
via the Nematrian
web function library by using a DistributionName of “student's t”.
Functions relating to a generalised version of this distribution including
additional location (i.e. shift) and scale parameters may be accessed by using
a DistributionName of “student's t3”, see also including
additional shift and scale parameters. For details of other supported
probability distributions see here.
NAVIGATION LINKS
Contents | Prev | Next