Special polynomials
[this page | pdf]
A (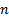
’th order) polynomial is a
function of the form 
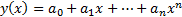where
the 
are
constant. They appear in many guises in mathematics. Whilst it is common to
focus on cases where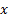 
is a real number, there are
attractions in extending their domain to relate to complex numbers,
with the also
then being allowed to be complex. For example, a polynomial of order 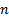always
then has 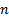(possibly not distinct)
roots, i.e. values of 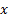where 
but
even if all the are
real some of these roots may be complex.
Several special types of polynomial have been widely
analysed, including:
-
Legendre polynomials
-
Chebshev polynomials
-
Hermite polynomials
-
Jacobi polynomials
-
Laguerre polynomials
These polynomials all appear in a natural way when we try to
approximate a functional form as follows.
Suppose we define a space of real or complex valued
(continuous) functions on the interval 
.
A natural ‘scalar product’ of two functions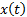 
and
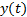is
then:
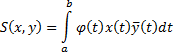
where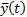 
is
the complex conjugate
of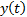 
and
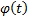is
a real continuous non-negative function (with at most finitely many zeros)
called the weight function for the given scalar product. If the 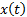and
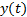are
limited to real functions then the definition simplifies to the folloing
(because the same formulae apply, but the complex
We may then, for example, define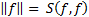 
.
If 
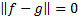then
and
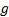are
then identical (if continuous) within the interval .
We also have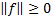 
for
all , so we can view as a good
approximation to 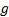if 
is
close to zero. Different weight functions then indicate where within the
interval we
most want the approximation to be accurate.
As with any vector space, we can define a basis of
orthogonal elements, 
(which
is here infinite dimensional) which in aggregate ‘span’ the entire vector
space, i.e. here the entire range of (continuous) functions defined on .
By orthogonal we mean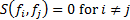 
.
The different special functions listed above provide natural orthogonal bases
for different weight functions:
Legendre: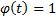 
and
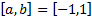(can
also be viewed as a special case of Jacobi with 
)
Jacobi: 
and
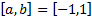
Chebyshev: the special case of the Jacobi with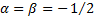 
which
means that they can be expressed in a simple analytical manner.
Laguerre: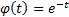 
and
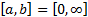
Hermite: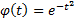 
and
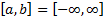
For example, the first few Legendre polynomials are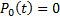 
,
,
,
,
…
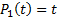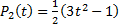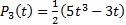The exact definition of each special polynomial type depends
on the ‘normalisation’ used. This is because if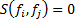 
then
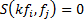for
any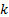 
. The usual normalisation
convention involves 
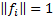for
all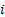 
.
In the financial world, the computation of many types of
risk measures is mathematically akin to a evaluating a particular integral. A
common way of carrying out numerical integration is to use an approach called
Gaussian quadrature. This is often implemented in a fashion that makes use of
some of the polynomials described above.