Refinements to definitions of the base
units of the SI system introduced on 20 May 2019
[this page | pdf | references | back links]
As explained in SI unit definitions
the kilogram used to be defined by reference to the mass of a specific material
artefact, i.e. the international prototype kilogram. The definitions of the
ampere, mole and candela then depended on the kilogram. Other base unit
definitions that had previously referred to specific material artefacts (e.g.
the metre) had been altered so that they refered directly to fundamental
constants of nature.
The 23rd (2007) and 24th (2011)
meetings of the CIPM included resolutions that aimed to redefine the kilogram
by reference to a fundamental constant of nature, and to redefine the mole and
candela to link more directly to such constants. Definitions were agreed by CIPM (2011)
but without some of the precise numerical values being then selected.
On 20 May 2019 the following exact numerical values were
adopted, linking all the fundamental SI units to constants of nature:
-
the ground state hyperfine splitting frequency of the caesium 133 atom is
exactly 9192631770 hertz
- the
speed of light in vacuum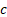 
is
exactly 299792458 metre per second
- the
Planck constant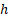 
is exactly
6.62607015 x 10-34 joule
second
- the
elementary charge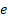 
is
exactly 1.602176634 x 10-19 coulomb
- the
Boltzmann constant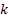 
is exactly
1.380649 x 10-23 joule per kelvin
-
the Avogadro constant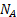 
is exactly
6.02214076 x 1023 reciprocal mole
-
the luminous efficacy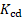 
of
monochromatic radiation of frequency 5.4 x 1014 is exactly 683 lumen
per watt
Such definitions do, of course, presuppose that fundamental
constants of nature really are fundamental, a topic that has been the subject
of some speculation over many years, see e.g. Barrow (2003).
These speculations generally revolve around what might be the case if dimensionless
constants such as the fine
structure constant varied across the universe. Changes merely in dimensional
physical constants, such as the speed of light are generally considered to be
less operationally meaningful as such a world would be observationally
indistinguishable from our world. A measurement system that is sometimes
referred to in such a context involves Planck units.
NAVIGATION LINKS
Contents | Prev | Next